The Human Body Temperature, Measured In Degrees Fahrenheit, Is (Approximately) Normally Distributed With Mean 98.6 And

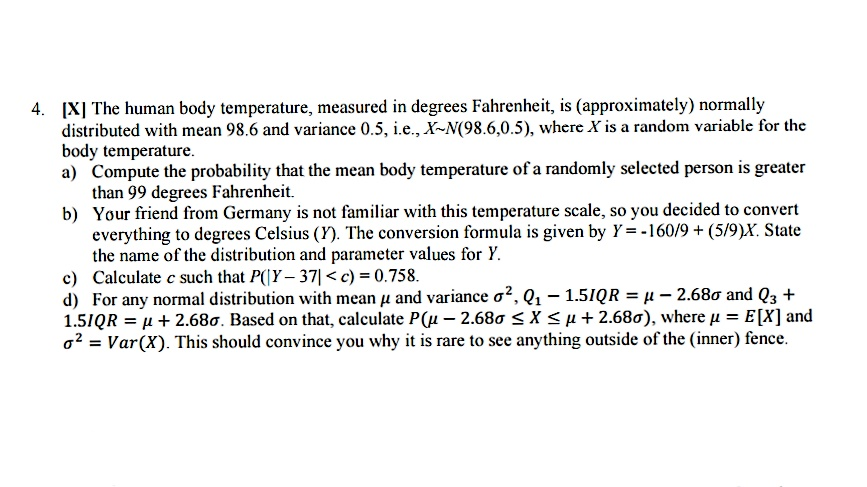
Transcribed image text: The human body temperature, measured in degrees Fahrenheit, is (approximately) normally distributed with mean 98.6 and variance 0.5, i.e., X~N(98.6, 0.5), where X is a random variable for the body temperature. a) Compute the probability that the mean body temperature of a randomly selected person is greater than 99 degrees Fahrenheit. b) Your friend from Germany is not familiar with this temperature scale, so you decided to convert everything to degrees Celsius (Y). The conversion formula is given by Y = -160/9 + (5/9)X. State the name of the distribution and parameter values for Y. c) Calculate c such that P(|Y-37| < c) = 0.758. d) For any normal distribution with mean mu and variance sigma^2, Q_1 - 1.5IQR = mu - 2.68 sigma and Q_3 + 1.5IQR = mu + 2.68 sigma. Based on that, calculate P(mu - 2.68 sigma lessthanorequalto X lessthanorequalto mu + 2.68 sigma), where n = E[X] and sigma^2 = Var(X). This should convince you why it is rare to see anything outside of the (inner) fence.